Maybe it's seven weeks without drinking, or maybe it's because this is how my mind works anyway, but I was thinking the other day about infinity.
Well, I say thinking, it crossed my mind. In the words of Bruce Dickinson, infinity really is hard to comprehend. I'm sure you don't need me to tell you that it's not an actual number
.
If someone gave you a billion biscuits once a second for twenty years... you'd still be nowhere close. Although you'd probably be sick of biscuits.
Anyway, the thing playing in my mind was that playground argument that most kids have done (including myself):
: I've seen Star Wars 10 times.
: I've seen Star wars 100 times.
: Well I've seen it infinity times
: I've seen it infinity-plus-one times
: aahahahah! you knob! there's no such thing as infinity-plus-one!
Because even child-logic manages to grasp that if infinity can't be defined as an actual number, you can't possibly add a number on to it. Can you? I think we can do the next best thing...
+++ +++
For today's exercise, we're going to create a ball. A red ball. We're going to create it out of whatever we can find to make it tough, because it's going to last forever. Don't ask how, it just will.

There's our ball. Date-stamped at midday today. This ball will outlast the earth, the galaxy and even the universe itself. Like I said, don't ask how, because for the purposes of this exercise, the ball is going to be chronolgically-infinite; and if this ball can't be, then neither can anything else.
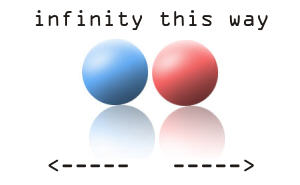
So, our ball has a fixed "start-point" in universal time. Before 12:00 today, it didn't exist. After 12:00 today, it did. 60 minutes after we make the red ball, we're going to make a blue one. Same materials, same properties, just a different colour.

This blue ball will also last forever. Same rules as above. We now have two balls (...quiet at the back), one an hour older than the other one; and that's the key.
If we come back to them tomorrow at 1pm, we can see how old they are:

As time is flowing smoothly, they've both existed at the same rate, and the red one is still an hour older than the blue one. Of course, at this point they're both roughly a day old. If we come back to them in a year...

And obviously, the red ball is still an hour older than the blue one. Of course, these are just snapshots of how they looked at any one point in time. The balls can't be said to be "infinite" yet, because they're both only a year(ish) old. This problem will be inherent in any snapshot we take because, as we identified earlier, we can't stop at "infinity" to take a picture - the number doesn't exist. But by now, I think you know where I'm heading with this.
So what we'll have to do next is make a statement, rather than a snapshot. Like I said, these balls will exist forever. We know that, because that's how we created them.

Although both balls a chronologically "infinite", the red ball will ALWAYS be one hour older than the blue one. Although the blue one will go on forever, the red one has been around longer. Can this be defined as "infinity-plus-one"?
Again, we're assuming there that infinity can be defined as a solid number. Although, to be fair, we're assuming that the balls will survive the end of the universe. As popular theory suggests the universe is 13.7 billion years old (which isn't a lot when you write it down), it seems silly to assume that it won't end at some point. And as we don't know what happened before the creation of the universe, we have no idea what will happen after it. Where do all the balls go?
Another way to look at it would be to suggest that as the balls have different-but-fixed starting points, they aren't truly infinite. Using the above exercise, any infinite object that was created before them would be older, and therefore "more infinite". Using that logic, the only infinte objects would be those that have always existed. Namely, objects that don't have a starting point, in the same way as the red and blue balls don't have an ending point. This would make the "original-infinite" objects older than the physical universe. If we could find one of these objects, we'd arguably have a way to find out what existed before the universe began.
But that's all wildly theoretical, of course. We haven't found an infinite object yet, and it seems a safer bet to assume that infinity can't exist, by its own definition.
...imagine the things I think about when I have been drinking.
Balls to it.
DISCLAIMERS:
• ^^^ That's dry, British humour, and most likely sarcasm or facetiousness.
• This is a personal blog. The views and opinions expressed here represent my own thoughts (at the time of writing) and not those of the people, institutions or organizations that I may or may not be related with unless stated explicitly.
DISCLAIMERS:
• ^^^ That's dry, British humour, and most likely sarcasm or facetiousness.
• This is a personal blog. The views and opinions expressed here represent my own thoughts (at the time of writing) and not those of the people, institutions or organizations that I may or may not be related with unless stated explicitly.

No comments:
Post a Comment